JEE Main 2023 Physics Solution-24th Jan Shift 1

Table of Contents

JEE Main 2023 Physics Solution-24th Jan Shift 1

Section-A

Q.1 From the photoelectric effect experiment, following observations are made. Identify which of these
are correct.
A. The stopping potential depends only on the work function of the metal.
B. The saturation current increases as the intensity of incident light increases.
C. The maximum kinetic energy of a photo electron depends on the intensity of the incident light.
D. Photoelectric effect can be explained using wave theory of light
Choose the cornet answer from the options given below:
Options 1. A, B, D only 2. B, C only 3. B only 4. A, C, D only
Answer- Option 3

Q.2 Given below are two statements
Statement I : If the Brewster's angle for the light propagating from air to glass is $\theta_B$ , then the
Brewster's angle for the light propagating from glass to ail is $\frac{\pi}{2}-\theta_B$
Statement II : The Brewster's angle for the light propagating from glass to air is $\tan^{-1}\mu_g$ where $\mu_g$
is the refractive index of glass.
In the light of the above statements, choose the correct answer from the options given below:
Options 1. Statement I is true but Statement II is false 2. Both Statement I and Statement II are false 3. Both Statement I and Statement II are true 4. Statement I is false but Statement II is true
Solution- Brewster's law for air to glass: $\tan{\theta_B}=\mu_g$
But, Brewster's law for glass to air: $\tan{\phi}=\frac{1}{\mu_g}$
∴ $\tan{\phi}=\frac{1}{\tan{\theta_B}}=\cot{\theta_B}$
or, $\tan{\phi}=\tan{\frac{\pi}{2}-\theta_B}$
or, $\phi=\frac{\pi}{2}-\theta_B$
Answer- Option 1

Q.3 Given below are two statements : one is labelled as Assertion A and the other is labelled as Reason R
Assertion A : Photodiodes are preferably operated in reverse bias condition for light intensity
measurement.
Reason R : The current in the forward bias is more than the current in the reverse bias for a p—n
junction diode.
In the light of the above statements, choose the correct answer from the options given below:
Options 1. A is false but R is true 2. A is true but R is false 3. Both A and R are true but R is NOT the correct explanation of A 4. Both A and R are true and R is the correct explanation of A
Answer- Option 3

Q.4 A circular loop of radius r is carrying IA. The ratio of filed at centre of circular loop and at a distance r form centre of the loop on its axis is:
Options 1. 1:3√2 2. 2√2:1 3. 1:√2 4. 3√2:2
Solution- Magnetic field at the centre of the loop $B_1=\frac{\mu_0 I}{2r}$
Magnetic field at dis r from the centre $B_2=\frac{\mu_0 I}{2(r^2+r^2)^{3/2}}$ = $\frac{\mu_0 I}{2\times 2\sqrt{2}}$
$B_1 : B_2 = \frac{\mu_0 I}{2r}:\frac{\mu_0 I}{4\sqrt{2}}$ = $\frac{1}{2}:\frac{1}{4\sqrt{2}}$ = 2√2:1
Answer- Option 2

Q.5 In $\vec{E}$ and $\vec{K}$ represent electric field and propagation vectprs of the EM waves in vacuum, then magnetic filed vector is given by: ( $\omega$ )
Options 1. $\vec{K}\times\vec{E}$ 2. $\frac{1}{\omega}(\vec{K}\times\vec{E})$ 3. $\omega (\vec{K}\times\vec{E})$ 4. $\omega(\vec{E}\times\vec{K})$
Answer- Option 2

Q.6 As shown in the figure, a network of resistors is connected to a battery of 24V with an internal resistance of 3Ω. The currents through the resistors $R_4$ and $R_5$ are $I_4$ and $I_5$ respectively. The values
of $I_4$ and $I_5$ are:

Options 1. $I_4=\frac{8}{5}A$ and $I_5=\frac{2}{5}A$ 2. $I_4=\frac{6}{5}A$ and $I_5=\frac{24}{5}A$ 3. $I_4=\frac{2}{5}A$ and $I_5=\frac{8}{5}A$ 4. $I_4=\frac{24}{5}A$ and $I_5=\frac{6}{5}A$
Solution- $R_{eq}=(R_1 || R_2)+R_3+(R_4 || R_5)+R_6 + r$ = $(\frac{1}{2}+\frac{1}{2})^{-1}+2+(\frac{1}{20}+\frac{1}{5})^{-1}+2+3$ = $1+2+\frac{20}{1+4}+5$ = 8+4 =12Ω
and total current I= $I_4 + I_5$ = $\frac{V}{R_{eq}}$ = $\frac{24}{12}$ = 2A
Also $\frac{I_4}{I_5}$ = $\frac{V/R_4}{V/R_5}$ = $\frac{R_5}{R_4}$ = $\frac{5}{20}$ = $\frac{1}{4}$ or, $I_5 = 4I_5$
∴ $I_4+4I_4=2$ or, $I_4 = \frac{2}{5}$ and $I_5=4\times \frac{2}{5}=\frac{8}{5}$
Answer- Option 3

Q.7 If two charges $q_1$ and $q_2$ are separated with distance 'd' and placed in a medium of dielectric constant k. What will be the equivalent distance between charges in air for the same electrostatic force?
Options 1. 5d√k 2. 2d√k 3. d√k 4. k√d
Solution- Force in that medium $F_k = \frac{1}{4\pi k\varepsilon_0}\frac{q_1q_2}{d^2}$ and Force in air $F_0 = \frac{1}{4\pi \varepsilon_0}\frac{q_1q_2}{r^2}$ .
But, $F_k=F_0$ or, $\frac{1}{4\pi k \varepsilon_0 }\frac{q_1q_2}{d^2}=\frac{1}{4\pi \varepsilon_0}\frac{q_1q_2}{r^2}$
or, $r^2 = kd^2$ or, r=√k d
Answer- Option 3

Q.8 The maximum vertical height to which a man can throw a ball is 136 m. The maximum horizontal distance upto which he can throw the same ball is:
Options 1. 192 m 2. 272 m 3. 136 m 4. 68 m
Solution- In projectile motion height $H=\frac{u^2}{2g}$ = 136 m
and range $R=\frac{u^2 \sin{(2\theta)}}{g}$ . ∴ $R_{max}=\frac{u^2 \sin{(2\times45\degree)}}{g}$ = $\frac{u^2}{g}$ = $2\frac{u^2}{2g}$ = 2H = 2×136 m = 272 m
Answer- Option 2

Q.9 Given below are two statements:
Statement I: The temperature of a gas is -73°C. When the gas is heated to 527°C, the root mean square speed of the molecules is doubled.
Statement II: The product of pressure and volume of an ideal gas will be equal to translational kinetic energy of the molecules.
In the light of the above statements, choose the correct answer from the options given below:
Options 1. Statement I is true but Statement II is false 2. Statement I is false but Statement II is true 3. Both Statement I and Statement II are true 4. Both Statement I and Statement II are false
Solution- For statement I: K.E at -73°C (or 200 K) = $\frac{3}{2}R.200=E_1$
K.E at 527°C (or 800 K) = $\frac{3}{2}R.800=E_2$
$\frac{E_1}{E_2}=\frac{\frac{3}{2}R.200}{\frac{3}{2}R.800}=\frac{1}{4}$ or, $\frac{\frac{1}{2}mc^2}{\frac{1}{2}mc^2}=1/4$ or, $\frac{c_1}{c_2}=\frac{1}{2}$ ∴ $c_2=2c_1$ [True]
For statement I: pV=nRT. Translational K.E. $\frac{3}{2}nRT=\frac{3}{2}pV$ [False]
Answer- Option 1

Q.10 As per given figure, a weightless pulley P is attached on a double inclined frictionless surface. The tension in the string (massless) will be (if g=10 m/s²)

Options 1. 4(√3+1) N 2. (4√3+1) N 3. 4(√3-1) N 4. (4√3-1) N
Solution-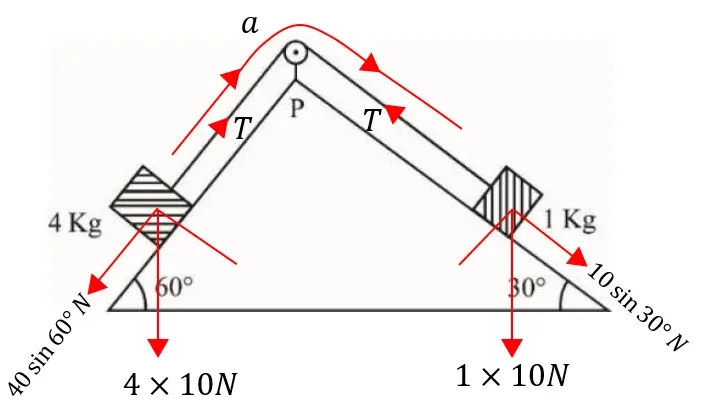
On the left inclined plane: $4.a=T-40\sin{60\degree}$ or, $a=\frac{T}{4}-5\sqrt{3}$
On the right inclined plane: $1.a=10\sin{30\degree}-T$ = $5-T$
Equating the value of $a$ : $\frac{T}{4}-5\sqrt{3}=5-T$
or, $\frac{5T}{4}=5(\sqrt{3}+1)$ ∴ T=4(√3+1) N
Answer- Option 1

Q.11 Consider the following radioactive decay process:
${}_{84}^{218}A\mathop{\rightarrow}\limits^{\alpha}A_1\mathop{\rightarrow}\limits^{\beta^-}A_2\mathop{\rightarrow}\limits^{\gamma}A_3\mathop{\rightarrow}\limits^{\alpha}A_4\mathop{\rightarrow}\limits^{\beta^+}A_5\mathop{\rightarrow}\limits^{\gamma}A_6$
The mass number and the atomic number are given by:
Option 1. 210 and 82 2. 210 and 80 3. 211 and 80 4. 210 and 84
Solution- Mass number after 2α emission: 218-2×4=210. Atomic number after 2α emission: 84-2×2=80.
Beta decay does not change the mass number but atomic number.
∴ Atomic number after $1\beta^+$ and $1\beta^-$ decay: 80+1-1=80
Answer- Option 2

Q.12 The weight of a body at the surface of earth is 18 N. The weight of the body at an altitude of 3200 km above the earth's surface is (given, radius of earth $R_e$ = 6400 km):
Options 1. 9.8 N 2. 19.6 N 3. 4.9 N 4. 8 N
Solution: $g' = \frac{g}{(1 + \frac{h}{R_e})^2}$ = $\frac{g}{(1 + \frac{3200}{6400})^2}$ = $\frac{4g}{9}$
or, $mg' = \frac{4mg}{9}$ = $\frac{4\times18}{9}$ N=8 N
Answer- Option 4

Q.13 A conducting circular loop of radius $\frac{10}{\sqrt{\pi}}$ cm is placed perpendicular to a uniform magnetic field of 0.5 T. The magnetic field is decreased to zero in 0.5 s at a steady rate. The induced emf in the
circular loop at 0.25 s is:
Options 1. 5 mV 2. 10 mV 3. 1 mV 4. 100 mV
Solution: Area of the loop- A= $\pi.(\frac{10}{\sqrt{\pi}})^2$ cm^2 = 100 cm² = 10^-2 m²
Find B as a function of t:
Now, $\frac{\Delta B}{\Delta t}$ = $\frac{0.5}{0.5}=1$
Flux $\phi=A.B$ or, emf= $\frac{\Delta\phi}{\Delta t}$ = $A.\frac{\Delta B}{\Delta t}$ = 10^-2.1 V= 10 mV
Answer: Option 2

Q.14 Two long straight wires P and Q carrying equal current 10A each were kept parallel to each other at 5 cm distance. Magnitude of magnetic force experienced by 10 cm length of wire P is $F_1$ . If distance between wires is halved and currents on them are doubled, force $F_2$ on 10 cm length of wire P will be:
Options 1. $\frac{F_1}{10}$ 2. $\frac{F_2}{8}$ 3. $10 F_1$ 4. $8 F_1$
Solution: Force per unit length, $F_1=\frac{\mu_0}{2\pi}\frac{I_1 I_2}{d}$
According to question new force, $F_2=\frac{\mu_0}{2\pi}\frac{2.I_1 2.I_2}{\frac{d}{2}}$ = $8\frac{\mu_0}{2\pi}\frac{I_1 I_2}{d}$ = $8 F_1$
Answer- Option 4

Q.15 Given below are two statements:
Statement I: An elevator can go up or down with uniform speed when its weight is balanced with the tension of its cable.
Statement II: Force exerted by the floor of an elevator on the foot of a person standing on it is more than his/her weight when the elevator goes down with increasing speed.
In the light of the above statements, choose the correct answer from the options given below:
Options 1. Statement I is false but Statement II is true 2. Statement I is true but Statement II is false 3. Both Statement I and Statement II are true 4. Both Statement I and Statement II are false
Answer- Option 2

Q.16 A modulating signal is a square wave, as shown in the figure.

If the carrier wave is given as c(t) = 2 sin (8лt) volts, the modulation index is:
Options 1. $\frac{1}{4}$ 2. 1 3. $\frac{1}{2}$ 4. $\frac{1}{3}$
Solution- modulation index= $\frac{V_m}{V_c}$ = ${1}{2}$
Answer- Option 3

Q.17 A travelling wave is described by the equation: y(x, t)= [0.05 sin (8x-4t)] m. The velocity of the wave is: [all the quantities are in SI unit]
Options 1. 0.5 ms^-1 2. 2 ms^-1 3. 8 ms^-1 4. 4 ms^-1Solution- General equation of travelling wave- $y = A\sin \frac{2\pi }{\lambda }\left(vt \pm x\right)$
Now, y(x, t)= $0.05 sin (8x-4t)$ = $-0.05 sin 8(\frac{t}{2}-x)$
Comparing both equation wave velocity 0.5 m/s
Answer- Option 1

Q.18 A 100 m long wire having cross-sectional area 6.25×10^-4 m² and Young's modulus is 10¹⁰ Nm^-2 is subjected to a load of 250 N, then the elongation in the wire will be:
Options 1. 6.25×10^-6m 2. 6.25×10^-3m 3. 4×10^-3m 4. 4×10^-4m
Solution- L=100m, A=6.25×10^-4 m², Y=10¹⁰ Nm^-2,F= 250 N
$Y=\frac{FL}{Al}$ or, $l=\frac{FL}{AY}$ = $\frac{250\times100}{6.25\times 10^{-4}\times 10^{10}}$ = 4×10^-3
Answer- Option 3

Q.19 1 g of a liquid is converted to vapour at 3×10⁵ Pa pressure. If 10% of the heat supplied is used for increasing the volume by 1600 cm³ during this phase change, then the increase in internal energy in the process will be:
Options 1. 4.32×10⁸ J 2. 4800 J 3. 4320 J 4. 432000 J
Solution- m=1g, P=3×10⁵ Pa, ΔV=1600 cm³= 1600×10^-6m³=16×10^-4m³
Work= PΔV= 3×10⁵×16×10^-4J =480J
10% heat =Work done =480J. ∴90% heat increases the internal energy i.e.ΔU= $\frac{480\times90}{10}$ J=4320J
Answer- Option 3

Q.20 Match List I with List II

LIST I	LIST II
A. Planck's constant (h)	I. [M¹L²T^-2]
B. Stopping Potential (V_s)	II. [M¹L¹T^-1]
C. Work function (Φ)	III. [M¹L²T^-1]
D. Momentum (p)	IV. [M¹L²T^-3A^-1]

Choose the correct answer from the options given below:
Options 1.A-III, B-IV, C-I, D-II 2. A-I, B-III, C-IV, D-I 3. A-II, B-IV, C-III, D-I 4. A-III, B-I, C-II, D-IV
Solution- h=momentum × distance}→[MLT^-1.L]=[M¹L²T^-1] ∴D-II
There is only one option having D-II.
Answer- Option 1

JEE Main 2023 Physics Solution-24th Jan Shift 1

Section-B

Q.21 $A block of a mass 2 kg is attached with two identical springs of spring constant 20 N/m each. The block is placed on a frictionless surface and the ends of the springs are attached to rigid supports (see figure). When the mass is displaced from its equilibrium position, it executes a simple harmonic motion. The time period of oscillation is <span class="katex-eq" data-katex-display="false">frac{\pi}{\sqrt{x}}</span> in SI unit. The value of x is$
A block of a mass 2 kg is attached with two identical springs of spring constant 20 N/m each. The block is placed on a frictionless surface and the ends of the springs are attached to rigid supports (see figure). When the mass is displaced from its equilibrium position, it executes a simple harmonic motion. The time period of oscillation is $\frac{\pi}{\sqrt{x}}$ in SI unit. The value of x is_______
Solution- If the block moves slightly to the right side, the spring of this side will be compressed and the other side of the spring will be stretched. Therefore, the forces applied by two spring will act from left to the right side direction to bring the block at equilibrium. This looks like a parallel combination of springs.
Therefore, $K_{eff}=2K$ =40N/m. Now, $\omega = \sqrt{\frac{K_{eff}}{m}}$ = $\frac{40}{2}$ = √20 = 2√5
or, $\frac{2\pi}{T}=2\sqrt{5}$ or, $T=\frac{\pi}{\sqrt{5}}$ ∴x=5

Q.22 In the circuit shown in the figure, the ratio of the quality factor and the band width is_______s
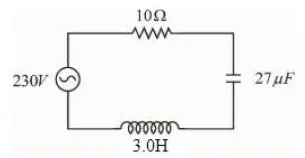Solution- $Q = \frac{1}{R}\sqrt{\frac{L}{C}}$ = $\frac{1}{10}\sqrt{\frac{3}{27\times 10^{-6}}}$ = $\frac{1}{10}.\frac{1}{3\times 10^{-3}}$ = $\frac{100}{3}$
$\Delta \omega = \frac{R}{L}$ = $\frac{10}{3}$
∴ $\frac{Q}{\Delta \omega}=\frac{\frac{100}{3}}{\frac{10}{3}}$ =10

Q.23 Vectors $a\widehat{i} + b\widehat{j} + \widehat{k}$ and $2\widehat{i} - 3\widehat{j} + 4\widehat{k}$ are perpendicular to each other when $3a +2b=7$ , the ratio of $a$ to $b$ is $\frac{x}{2}$ . The value of x is____
Solution- $(a\widehat{i} + b\widehat{j} + \widehat{k}).(2\widehat{i} - 3\widehat{j} + 4\widehat{k})=0$
or, $2a-3b+4=0$ or, $2a-3b=-4$ (i)×3
and $3a +2b=7$ (ii)×2
We get,
$6a - 9b = - 12$
$\underline{6a +4b = 14}$
or, -13b=-26 [বিয়োগ করে]
or, b=2
আবার, $3a +2\times 2=7$ or, $a=1$

Q.24 A hole is drilled in a metal sheet. At 27°C, the diameter of hole is 5 cm. When the sheet is heated to
177°C, the change in the diameter of hole is d×10^-3 cm. The value of d will be _______ if coefficient of linear expansion of the metal is 1.6×10^-5/°C.
Solution- D=5 cm, $t_1=27\degree C$ , $t_2=177\degree C$ , $α=1.6×10^{-5}/°C$
change in diameter = $D\alpha \Delta t$ = $5×1.6×10^{-5}\dot(177-27)$ cm = $8×10^{-5}×150$ cm = 12×10^-3 cm

Q.25 Assume that protons and neutrons have equal masses. Mass of a nucleon is 1.6×10⁻²⁷ kg and
radius of nucleus is 1.5×10^-15 A^1/3 m. The approximate ratio of the nuclear density and water density is n×10¹³. The value of n is_______.
Solution- Mass of nucleon = $m_n$ = 1.6×10⁻²⁷ kg, R= 1.5×10^-15 A^1/3 m where, A is atomic number, density of water 10³ kg/m³
Nuclear density = $\frac{A m_n}{\frac{4}{3}πR^3}$ . Now, according to question-
$n×10^{13}=\frac{\frac{A m_n}{\frac{4}{3}πR^3}}{10^3}$
or, $n×10^{13}=\frac{A m_n}{\frac{4}{3}πR^3×10^3}$
or, $n×10^{13}=\frac{A ×1.6×10^{−27}}{\frac{4}{3}π(1.5×10^{-15})^3×A×10^3}$
or, $n×10^{13}≈\frac{1.6 \times 10^{- 30}}{4 \times 1.5 \times 1.5^2 \times 10^{- 45}}$ ( $\frac{4}{3}π≈4$ )
or, $n×10^{13}≈\frac{10^{15}}{4 \times 2.25}$ (1.6≈1.5)
or, $n×10^{13}=\frac{100×10^{13}}{9}$ ≈11×10¹³
∴n=11

Q.26 A spherical body of mass 2 kg starting from rest acquires a kinetic energy of 10000 J at the end of 5^th second. The force acted on the body is _______ N.
Solution- $\frac{1}{2}mv^2=10000$ or, $\frac{1}{2}×2×v^2=10000$ ∴v = 100
F = ma = $m.\frac{v-u}{t}$ = $2.\frac{100-0}{5}$ = 20 N

Q.27 As shown in the figure, a combination of a thin plano concave lens and a thin plano convex lens is used to image an object placed at infinity. The radius of curvature of both the lenses is 30 cm and refraction index of the material for both the lenses is 1.75. Both the lenses are placed at distance of 40 cm from each other. Due to the combination, the image of the object is formed at distance x =_________cm, from concave lens.
$As shown in the figure, a combination of a thin plano concave lens and a thin plano convex lens is used to image an object placed at infinity. The radius of curvature of both the lenses is 30 cm and refraction index of the material for both the lenses is 1.75. Both the lenses are placed at distance of 40 cm from each other. Due to the combination, the image of the object is formed at distance x =_________cm, from concave lens.$
Solution- $\frac{1}{f} =(\mu -1)(\frac{1}{r_1}-\frac{1}{r_2})$
Focal length of plano concave lens (L₁): f₁
∴ $\frac{1}{f_1}= (1.75-1)(\frac{1}{\infty}- \frac{1}{30})$ = $- 0.75\times\frac{1}{30}$ = $-\frac{75}{100} \times \frac{1}{30}$ = $- \frac{1}{40}$ or, $f_1=-40$
Focal length of plano concave lens (L₂): f₂
∴ $\frac{1}{f_2}= (1.75-1)(\frac{1}{30}- \frac{1}{\infty})$ = $0.75\times\frac{1}{30}$ = $\frac{75}{100}\times \frac{1}{30}$ = $\frac{1}{40}$ or, $f_2=40$
Since, parallel ray are falling on L₁ lens, a virtual image will form to the left side of this lens at distance of 40 cm.
Now, for L₂ lens: object distance = (40+40) cm = 80 cm.
$\frac{1}{v}-\frac{1}{u}=\frac{1}{f_2}$
or, $\frac{1}{v}-\frac{1}{-80}=\frac{1}{40}$
or, $\frac{1}{v}=\frac{1}{40}-\frac{1}{80}$ = $\frac{1}{80}$
∴ $v=80$ cm
∴ Distance of image and object from plano concave lens = (40+80) cm= 120 cm

Q.28 Solid sphere A is rotating about an axis PQ. If the radius of the sphere is 5 cm then its radius of gyration about PQ will be √x cm. The value of x is ______.

Solution- $Mx^2=\frac{2}{5}Mr^2+M.10^2$
or, $x^2=\frac{2}{5}M5^2+10^2$
or, $x^2=100+100$
∴ $k=\sqrt{110}$

Q.29 A stream of a positively charged particles having $\frac{q}{m}=2×10^{11}\frac{C}{kg}$ and velocity $\overrightarrow{v_0} = 3 \times 10^7\widehat{i}$ m/s is deflected by an electric field $1.8\widehat{j}$ kV/m. The electric field exists in a region of 10 cm along x direction. Due to the electric field, the deflection of the charge particles in the y direction is _______ mm.
Solution- $\overrightarrow{F} = q\overrightarrow{E}$ = $q×1.8\widehat{j}$ kV/m = $q×1.8×10^3\widehat{j}$ V/m
$\overrightarrow{a} = \frac{\overrightarrow{F}}{m}$ = $\frac{q}{m}×1.8×10^3\widehat{j}$ m/s² = $2×10^{11}×1.8×10^3\widehat{j}$ m/s²
$10\widehat{i}\;cm = 0.1\widehat{i}\;m$ distance is travelled within $\frac{0.1}{v_0}$ s = $\frac{0.1}{3 \times 10^7}$ s = $\frac{10^{- 8}}{3}$ s
In this time, distance travelled by the particle along y axis is $\frac{1}{2} \times 2 \times 10^{11} \times 1\ldotp 8 \times 10^3 \times (\frac{10^{- 8}}{3})^2$ cm = $1\ldotp 8 \times 10^{14} \times \frac{10^{- 16}}{9}$ cm= $2×10^{-3}$ m = 0.02 mm

Q.30 A hollow cylindrical conductor has length of 3.14 m, while its inner and outer diameters are 4 mm and 8 mm respectively. The resistance of the conductor is n×10^-3Ω. If the resistivity of the material is 2.4×10^-8Ωm. The value of n is __________.
Solution- Inner and outer radii are 2 mm and 4 mm respectively.
Area of hollow cross section A =π(R²-r²) =3.14(4²-2²) mm² =3.14×(4+2)(4-2)×(10^-3)²m² =3.14×6×2×10^-6m² =3.14×12×10^-6m²
Resistance R= $\rho \frac{l}{A}$ = $2.4×10^{-8}\frac{3.14}{3.14×12×10^{-6}}Ω$ =0.2×10^-2 =2×10^-3Ω

JEE Main 2023 Physics Solution-24th Jan Shift 1